The raw material and manufacturing cost formed individually 70% and 30% of the total cost and the profit percentage is 14.28% of the raw material. If the cost of raw material increase by 20% and the cost of manufacturing is increased by 40% and the selling price is increased by 80%, then the new profit percentage is :
(a) 57\displaystyle\frac{1}{7}%
(b) 65.8%
(c) 60%
(d) Can’t be determined

Ans: (a) 57\displaystyle\frac{1}{7}%

Explanation:

Let, total cost = 100x
Raw material cost = 70x
Manufacturing cost = 30x

Profit = 70x × 14.28% = 70x × \displaystyle\frac{1}{7} = 10x

If you remember some fraction to to percentage conversion and vice versa, things becomes so easy.

Selling price = cost price + profit
\qquad\qquad\quad= 100x + 10x
\qquad\qquad\quad= 110x

New,
Raw material cost = 70x × \displaystyle\frac{120}{100} = 84x

Manufacturing cost = 30x × \displaystyle\frac{140}{100} = 42x

∴ Total cost = 126x

Selling price = 110x × \displaystyle\frac{180}{100} = 198x

Profit = 198x – 126x = 72x

∴ Profit % = \displaystyle\frac{72\text{x}}{126\text{x}} × 100 = 57\displaystyle\frac{1}{7}%

Aliter

In the exam we could avoid writing so much text if we solve in the following way.

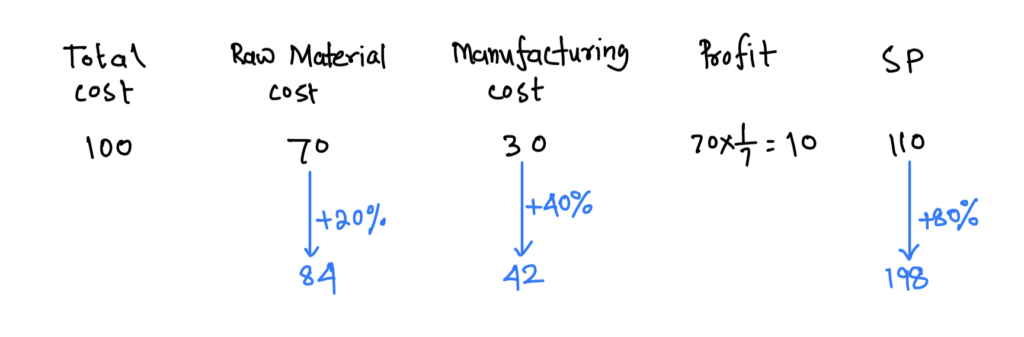
New cost = 84 + 42 = 126
Profit = 198 – 136 = 72

P% = \displaystyle\frac{72}{126}\times 100 = 57\displaystyle\frac{1}{7}\%